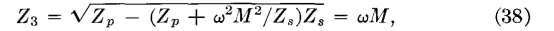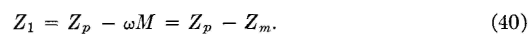Transformer Equivalent Networks

Figure 15. Circuits for studying the compensation theorem.

Figure 16. Circuit for determining maximum power transfer relations.

Z_12sc = Z_p + (ωM)²/Z_s. Thus, it is possible from these three measurements to determine both the important constants of a transformer, and the equivalent T network.

If the values just discussed are inserted in equations 21, 20, and 19 in the order given,

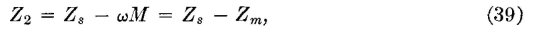
and

The reason that ωM = Z_m, where Z_m is called the mutual impedance, can be explained in the following manner. From Fig. 17, when the secondary is open, the voltage E₃₄ across the secondary is equal to the I_pZ₃ voltage drop. Thus,

Z₃ = E₃₄/I_p = ωMI_p/I_p = ωM =

Z_m, the term mutual impedance being used because, for the actual transformer of Fig. 17, the secondary voltage #34 is being divided by the primary current I_P.

Transformers are used in communication circuits for impedance matching (page 68). For such purposes, an ideal transformer is assumed. Such a transformer will change the magnitude of the load impedance without altering the angle of the load impedance and introduces no losses into the circuit. An ideal transformer is assumed in making possible maximum power transfer under the conditions of the second maximum power transfer theorem.