Index of Refraction of a Plate

Let P (fig. 37) be a point in a medium of refractive index μ, and let a small pencil of rays diverging from this point fall directly on the plane-bounding surface of the medium and emerge into air.

Let A be the point at which the axis of the pencil emerges, and Q a point on PA, such that AP = μAQ; then the emergent pencil will appear to diverge from Q, and if we can measure the distances AP and AQ we can find μ. To do this, suppose we have a portion of a transparent medium in the form of a plate, and a microscope, the sliding tube of which is fitted with a scale and vernier or at least a pointer, so that any alteration in the position of the object-glass when the microscope is adjusted to view objects at different distances may be measured.

Place under the object-glass a polished disc of metal with a fine cross engraved on it, and bringing it into the centre of the field, focus the microscope to view the cross and read the scale. Repeat the observation several times, taking the mean. Now bring between the metal plate and the object-glass the transparent plate, which, of course, must not be of more than a certain thickness. One surface of the plate is in contact with the scratch on the metal, which thus corresponds to the point P: the emergent rays therefore diverge from the point Q, and in order that the scratch may be seen distinctly through the plate, the microscope will require to be raised until its object-glass is the same distance from Q as it was originally from P. Hence, if we again focus the microscope to see the cross, this time through the plate, and read the scale, the difference between the two readings will give us the distance PQ. Let us call this distance a, and let t be the thickness of the plate, which we can measure by some of the ordinary measuring apparatus, or, if more convenient, by screwing the microscope out until a mark, made for the purpose, on the upper surface of the plate comes into focus, and reading the scale on the tube.

We thus can find PA = t, PQ = a. But we have

and

A modification of this method is useful for finding the index of refraction of a liquid.

Suppose the liquid to be contained in a vessel with a fine mark on the bottom.

Focus on the mark through the liquid, and then on a grain of lycopodium dust floating on the surface. If the depth be d₁ the difference between the readings gives us d₁/μ; let us call this difference a. Then

Now add some more liquid until the depth is d₁+d₂. Focus on the mark again, and then a second time on the floating lycopodium which has risen with the surface; let the difference between these two be b; then

But the difference between the second and fourth reading, that is to say, of the two readings for the lycopodium grains is clearly the depth of liquid added, so that from these two readings d₂ is obtained, and we have

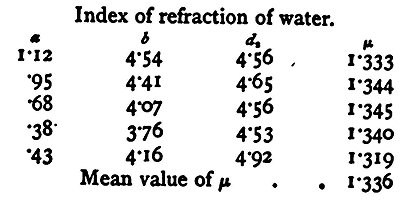
Experiment. - Determine the index of refraction (1) of the given plate and (2) of the given liquid.

Enter results thus: -