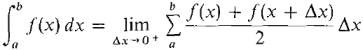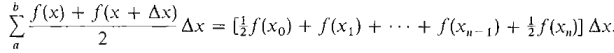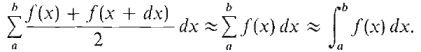The ebook Elementary Calculus is based on material originally written by H.J. Keisler. For more information please read the copyright pages.

Home Integral Numerical Integration Trapezoidal Approximation
 
del.icio.us Reddit Facebook StumbleUpon MySpace Twitter Search the VIAS Library  |  Index

Trapezoidal Approximation

We thus make the definition:

DEFINITION

Let Δx = (b - a)/n evenly divide b - a. Then by the trapezoidal approximation to the definite integral 04_integration-436.gif we mean the sum

04_integration-439.gif

The Trapezoidal Approximation of an integral 04_integration-437.gif can be computed very efficiently on most hand calculators. First compute the sum

½f(x0) + f(x1) + f(x2) + ... + ½ f(xn)

by cumulative addition. Then multiply this sum by Δx to obtain the Trapezoidal Approximation.

THEOREM 1

For a continuous junction f on [a, b], the trapezoidal approximation approaches the definite integral as Δx → 0+, that is,

04_integration-438.gif

PROOF

Comparing the formulas for the trapezoidal approximation and the Riemann sum, we see that

04_integration-445.gif

For dx positive infinitesimal, the extra term

(½f(xH)-½f(x0))dx

is infinitely small. It follows that

04_integration-440.gif
Home Integral Numerical Integration Trapezoidal Approximation

Last Update: 2010-11-26