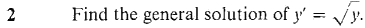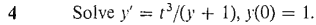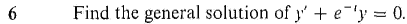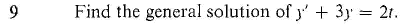Extra Problems (Differential Equations)

11            A population has a net birthrate of 2% per year and a constant net immigration rate of 50,000 per year. At time t = 0, the population is one million. Find the population y as a function of t.

12            Repeat Problem 11 for a net immigration rate of -50,000 per year (that is, emigration exceeds immigration by 50,000 per year).

13            Show that the initial value problem y' = cos (y² + t), y(0) = 1, has a unique solution for 0 ≤ t < ∞.

14            Show that the initial value problem y' = 1/(2 + sin y), y(0) = 1, has a unique solution for 0 ≤ t < ∞.

15            Find the general solution of y" - 5y' + 4y = 0.

16            Find the general solution of y" + 400y = 0.

17            Find the general solution of y" - 4y' + Sy = 0.

18            Find the general solution of y" - 14y' + 49y = 0.

19            Solve y" + 4y' - 5y = 0, y(G) = 0, y'(0) = 1.

20            Solve y" - 20y' + 100y = 0, j(0) = 1, y'(0) = 0.

21            A mass-spring system mx" + bx' + kx = 0 has mass m = 2 gm and constants b = 6 and k = 5. At time t = 0, its position is x(0) = 10 and its velocity is x'(0) = 0. Find its position x as a function of t.

22            Work Problem 21 if the system is subjected to constant external force of 3 dynes.

23            Find the general solution of y" - 5y' + 4y = 2 + t.

24            Find the general solution of y" + 400y' = e^t.

25            Find the general solution of y" - 4y' + 8y = cos t.

26            Find the general solution of y" - 14y' + 49y = t².

27            Solve y" + 4y' - 5y = 26 sin t, y(0) = 0, y'(0) = 0.

28            Solve y" - 20y' + 100 = e^10t, y(0) = 0, y'(0) = 0.