Practical Details of Manipulation

All delicate balances are fitted with a long pointer fixed to the beam, the end of which moves over a scale as the beam turns.

The middle point of this scale should be vertically below the fulcrum of the beam, and if the balance be in perfect adjustment, when the scale pans are empty and the beam free, the end of the pointer will coincide with the middle division of the scale. This coincidence, however, as we have seen, is not rigorously necessary.

To weigh a body we require to determine first at what point of the scale the pointer rests when the pans are empty. We then have to put the body to be weighed in one pan and weights in the other, until the pointer will again come to rest opposite to the same division of the scale. The weight of the body is found by adding up the weights in the scale pan.

We shall suppose that the weights used are grammes, decigrammes, &c.

The weights in the boxes usually supplied are some of them brass and the others either platinum or aluminium.

The brass weights run from 1 gramme to 50, 100 or 1000 grammes in different boxes.

We may divide the platinum and aluminium weights into three series:

The first includes, 0.5, 0.2, 0.1 gramme
The second 0.05, 0.02, 0.01 gramme
The third 0.005, 0.002, 0.001 gramme

that is, the first series are decigrammes, the second centigrammes, and the third milligrammes.

The weights should never be touched with the fingers; they should be moved by means of the small metal pliers provided for the purpose. In the larger boxes a brass bar is provided for lifting the heavier weights.

When the balance is not being used, the beam and the scale pans do not rest on the knife-edges but on independent supports provided for them. The balance is thrown into action by means of a key in the front of the balance case. This must always be turned slowly and carefully, so as to avoid any jarring of the knife-edges from which the beam and scale pans hang.

When it is necessary to stop the beam from swinging, wait until the pointer is passing over the middle of the scale, and then turn the key and raise the frame till it supports the beam. The key must not be turned, except when the pointer is at the middle of the scale; for if it be, the supporting frame catches one end of the beam before the other, and thus jars the knife-edges.

The weights or object to be weighed when in the scale pans must never be touched in any way while the beam is swinging; thus, when it is required to change the weights, wait until the pointer is passing across the middle point of the scale, turn the key, and fix the beam, then move the weights from the scale pan.

In the more delicate balances, which are generally enclosed in glass cases, it will be seen that the length of each arm of the beam is divided into ten parts.

Above the beam, and slightly to one side of it, there is a brass rod which can be moved from outside the balance case. This rod carries a small piece of bent wire, which can, by moving the rod, be placed astride the beam. This piece of wire is called a 'rider.' The weight of the rider is usually one centigramme.

Let ACB, fig. 8, be the beam, C being the fulcrum; the divisions on the arm are reckoned from C.

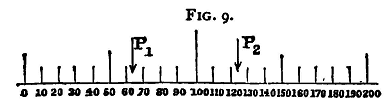
Suppose now we place the centigramme rider at division 1, that is one-tenth of the length of the arm away from the fulcrum, it will clearly require one-tenth of its own weight to be placed in the scale pan suspended from B, to balance it. The effect on the balance-beam of the centigramme rider placed at division 1, is the same as that of a weight of ¹/₁₀ centigramme or 1 milligramme in the pan at A. By placing the rider at division 1, we practically increase the weight in the pan at A by 1 milligramme. Similarly, if we place the rider at some other division, say 7, we practically increase the weight in A by 7 milligrammes.

The rider should not be moved without first fixing the balance beam.

Thus without opening the balance-case we can make our final adjustments to the weights in the scale pan by moving the rider from outside.

The object of the case is to protect the balance from draughts and air currents. Some may even be set up inside the case by opening it and inserting the warm hand to change the weights; it is therefore important in delicate work to be able to alter the weight without opening the case.

We proceed now to explain how to determine at what point of the graduated scale the pointer rests when the pans are empty. If the adjustments were quite correct, this would be the middle point of the scale. In general we shall find that the resting-point is somewhere near the middle.

We shall suppose for the present that the stand on which the balance rests is level. This should be tested by the spirit-level before beginning a series of weighings, and if an error be found, it should be corrected by moving the screw-feet on which the balance-case rests.

We shall find that the balance when once set swinging will continue in motion for a long period. The pointer will oscillate across the scale, and we should have to wait for a very long time for it to come to rest

We require some method of determining the resting-point from observations of the oscillations.

Let the figure represent the scale, and suppose, reckoning from the left, we call the divisions 0, 10, 20, 30,.....

A little practice enables us to estimate tenths of these divisions.

Watch the pointer as it moves; it will come for a moment to rest at pl suppose, and then move back again. Note the division of the scale, 63, at which this happens.¹ The pointer swings on past the1 resting-point, and comes to instantaneous rest again in some position beyond it, as P₂, at 125 suppose.

Now if the swings on either side of the resting-point were equal, this would be just half-way between these two divisions, that is at 94; but the swings gradually decrease, each being less than the preceding. Observe then a third turning point on the same side as the first, P₃ suppose, and let its scale reading be 69.

Take the mean 66, between 69 and 63. We may assume that this would have been the turning-point on that side at the moment at which it was 125 on the other, had the pointer been swinging in the opposite direction. Take the mean of the 125 and 66, and we have 95.5 as the value of the resting-point.

Thus, to determine the resting point:

Observe three consecutive turning points, two to the left and one to the right, or vice versd. Take the mean of the two to the left and the mean of this and the one to the right; this gives the resting-point required.

The observations should be put down as below.

We may, if we wish, observe another turning-point to the right, 120 suppose; then we have another such series.

Proceeding thus we get a set of determinations of the resting-point, the mean of which will give us the true position with great accuracy.

Having thus found the resting point with the pans empty, turn the key or lever, and fix the beam; then put the object to be weighed in one scale pan. Suppose it to be the left-hand, for clearness in the description. Then put on some weight, 50 grammes say, and just begin to turn the key to throw the balance into action. Suppose the pointer moves sharply to the left, 50 gms. is too much. Turn the key back, remove the 50 and put on 20; just begin to turn the key; the pointer moves to the right, 20 is too little. Turn the key back, and add 10; the pointer still moves to the right; add 10 more, it moves to the left; 40 is too much. Turn the key back, remove the 10 and add 5. Proceed in this way, putting on the weights in the order in which they come, removing each weight again if the pointer move sharply to the left, that is, if it be obviously too much, or putting on an additional weight if the pointer move to the right

There is no necessity to turn the key to its full extent to decide if a weight be too much or too little until we get very nearly the right weight; the first motion of the pointer is sufficient to give the required indication.

It saves time in the long run to put on the weights in the order in which they come in the box.

Caution. - The beam must always be fixed before a weight is changed.

Suppose now we find that with 37.68 grammes the pointer moves to the right, showing the weight too little, and that with 37.69 the motion is to the left, showing that it is too much. Close the balance-case, leaving on the lighter weight, 37.68 grammes. Turn the key, and notice if the pointer will swing off the scale or not Suppose it is quite clear that it will, or that the resting-point will be quite at one end near the division 200. Fix the beam, and put on the rider say at division 2. This is equivalent to adding 0.002 gm. to the weights in the scale pan, so that the weight there may now be reckoned as 37.682 gms. Release the beam, and let it oscillate, and suppose that this time the pointer remains on the scale.

Read three turning-points as before.

Thus we find that with no weights in the scale pans, the resting-point is 95.5 - we may call this 96 with sufficient accuracy - while, with the object to be weighed in the left pan, and 37.682 grammes in the right, the resting-point is 134.

Hence 37.682 gms. is too small, and we require to find what is the exact weight we must add to bring the resting-point from 134 to 96, that is, through 38 divisions of the scale.

To effect this, move the rider through a few divisions on the beam, say through 5; that is, place it at division 7. The effective weight in the scale pan is now 37.687 gms.; observe as before.

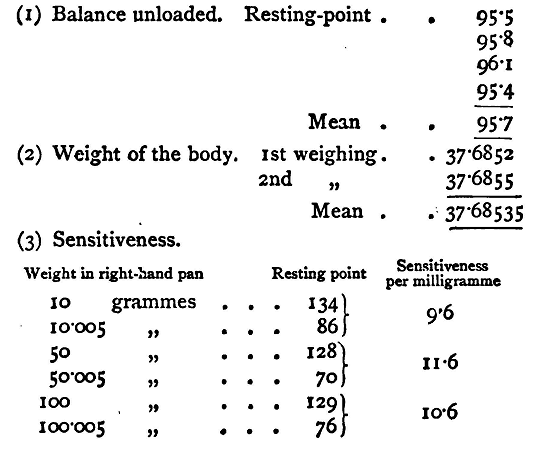
The addition of 0.005 gramme has moved the resting-point from 134 to 74; that is, through 60 divisions.

We have then to determine by simple proportion what weight we must add to the 37.682 in order to move the resting-point through the 38 divisions; that is,, from 134 to 96. The weight required is ³⁸/₆₀ x 0.005 or 0.00316 gm. If then we add 0.00316 gm. to the 37.682, the resting-point will be 96, the same as when the scale pans were empty.

Thus the weight of the body is 37.68516 gms. We have not been working with sufficient accuracy to make the last figure at all certain; we will therefore discard it, and take the weight as 37.6852 grammes (p. 37).

One or two other points require notice.

In each case we have supposed the pointer to swing over from 60 to 70 divisions; this is as large a swing as should be allowed.

We have supposed the resting point, when the balance was unloaded, to lie between those for the two cases in which the load was 37.682 and 37.687; the weights should always be adjusted so that the like may be the case.

We have supposed that the weight for which we first observe the swing is too small. It is more convenient that this should be so; it is not absolutely necessary: we might have started from the heavier weight, and then moved the' rider so as to reduce the weight in the right-hand pan.

We must be careful to make no mistake as to the weights actually in the scale pan. It is generally wise for beginners to add them up as they rest on the pan, putting down each separately, grouping those weights together which belong to each separate digit, thus arranging them in groups of grammes, decigrammes, centigrammes, and milligrammes, and then to check the result by means of the vacant places left in the box.

When the weighing is completed see that the weights are replaced in their proper positions in the box, and that the beam is not left swinging.

We shall in future refer to this method of weighing as the ' method of oscillations.'

The alteration produced in the position of the resting point for a given small addition to the weights in the pan is called, as we have seen, the sensitiveness of the balance for that addition (p. 86).

Thus in our case the resting-point was altered by 60 for an addition of 0.005 gramme.

The sensitiveness, then, is ⁶⁰/₅ or 12 per milligramme.

The load in the pans in this case was nearly 38 grammes.

We should find by experiment that the sensitiveness depends slightly on the load in the pans. (See p. 86.)

Experiments.

(1) Determine the position of the resting-point four times when the balance is unloaded.

(2) Weigh the given body twice.

(3) Determine the sensitiveness for loads of 10, 50, and 100 gms.

Enter results thus: -

1	A small mirror is usually fixed above the scale, the planes of the two being parallel. When making an observation the observer's eye is placed so that the pointer exactly covers its own image formed in the mirror; any error due to parallax is thus avoided.