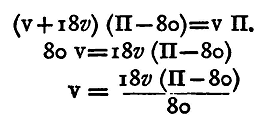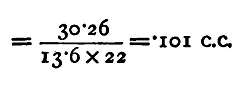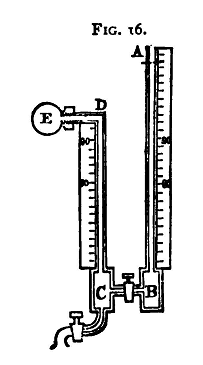
The Volumenometer

This apparatus consists of two glass tubes placed in a vertical position against two scales. The tubes are cemented into iron blocks, and connected together at the bottom by a short piece of tube with a tap.

One of the vertical tubes, C D (fig. 16), has a tap at the bottom, and at the top an elbow with a screw, by means of which a small flask, D E, can be fastened on.

The instrument is supported on three screws, by means of which it can, with the aid of a spirit-level, be put level.

The whole apparatus should stand in a wooden tray, which serves to catch any mercury that may unavoidably be spilt.

(1) To test Boyle's Law, viz. if V be the Volume and p the Pressure of a Mass of Gas at Constant Temperature, then Vp is Constant.

First level the apparatus.

The tubes require filling with mercury. They must be made quite clean and dried thoroughly by passing dry air through them.

Remove the flask, E. Open the tap between B and C and, fixing a glass funnel on at A, pour mercury down. A certain quantity of air will get carried down with the mercury. This can generally be removed by tilting the apparatus, or by means of an iron wire. The mercury must be perfectly clean and pure, otherwise it will stick to the glass and cause endless trouble.

Let us suppose that the level of the mercury is the same in both tubes, standing at division 90, say. (The smaller divisions of the scale at the Cavendish Laboratory are millimetres; the numbered divisions are centimetres.)

Screw on the flask gently, applying grease⁽¹⁾ to the washer to make it airtight.

Take care that the level of the mercury in the tubes is not altered by screwing on the flask. This sometimes happens from the heat of the hand causing the air inside to expand; then when the hand is removed the air inside contracts and the level of the mercury is altered. This may be avoided by screwing up the flask till it is almost tight, then waiting a little till it acquires the temperature of the room, and then completing the operation of screwing.

Let V denote the volume of the air in the flask and upper portion of the tube D C above the mercury - that is, down to the graduation 90. Since the mercury is at the same height in both limbs, the pressure of the air in the flask is the same as the pressure of the atmosphere, which we will suppose to be that due to Πmm. of mercury. Let us suppose also that the volume of a length of the tube of 1 cm. is v cubic centimetres.

Now open the tap C and let some mercury run out into a beaker.

The level of the mercury will sink in both tubes, but it will be found to be lower in A B than in C D.

Let us suppose that it stands at 72 in C D and 64 in A B.

Then the difference in pressure in the two tubes is that due to 72 - 64 cm. or 80 mm. of mercury.

The pressure in A B is Φ, that in D c then is Φ - 80.

The volume of the air has been increased in consequence of the diminution of pressure, by the volume of a length of 18 centimetres of the tube, and the volume of 18 centimetres is 18v; the new volume is therefore (V+18v).

Thus, applying Boyle's law,

If we know v, this gives us a value for V on the assumption that Boyle's law is true.

We have yet to determine v, the volume of 1 centimetre of length of the tube.

This may be done previously to the experiment described above in the following manner.

Fill the tubes with mercury and close the tap between B and c. Open the tap at C and allow mercury to run out from the tube C D, noting the division, 90 say, from which it begins to run. Allow the mercury to run out for 20 or 25 centimetres (suppose till the level reaches 68), and weigh the mercury. Let the weight be 30.26 grammes. The density of mercury is 13.6 gm. per c.c. Thus the volume is 30.26/13.6 c.c.

This is the volume of a length of 22 cm.; thus v, the volume of 1 cm.,

The determination of the volume of a centimetre of the tube should be made three times for different parts in order to calibrate the tube, §8.

Let us suppose that Φ, the height of the barometer, is 760. Then we find

Now open the stop-cock at C again, let some more mercury run out, and observe the difference of levels as before. Calculating in the same way, we can get another value for the volume V, and these two or more values thus obtained should of course be the same, provided that Boyle's law is true. Thus a comparison of the values of V obtained in this manner affords a verification of Boyle's law. There is a liability to considerable error in the observations, in consequence of alterations in the temperature of the air in the flask. These must be guarded against as carefully as possible, and, if greater accuracy be required, allowed for.

Before taking any of the readings to determine the difference of pressure, it is well to wait for a few minutes after the mercury has been run out, and see if the level of the mercury remains the same. If it does, we may feel sure that there is no leakage from the joint at D.

(2) To determine by means of the Volumenometer the Density of a Solid.

This method is applicable in the case of solids soluble in or affected by water. The solid should be broken into fragments sufficiently small to go into the flask D E.

Weigh the solid.

Determine as above the volume of the flask and portion of the tube C D to the division 90.

Introduce the solid into the flask, and again determine the volume of the flask and tube C D to the same division 90. The difference between the two volumes is of course the volume of the solid.

The density of the solid will be given by dividing the mass in grammes by the volume in cubic centimetres. The volume of the solid should be considerable; it should nearly fill the flask.

Experiments

(1) Test Boyle's law by measuring the volume of a small flask or test-tube attached to the volumenometer.

(2) Determine by means of the volumenometer the density of the given light solid. Enter results thus:

(1)	A very good compound for this and similar purposes is easily made by melting together equal quantities of pure vaseline and bees' wax. It should when cold be tolerably firm, and is always ready for use.