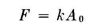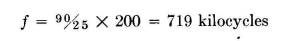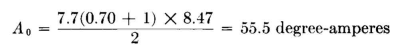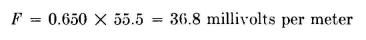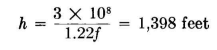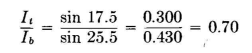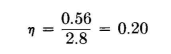Degree-Amperes and Field Strength

Author: Edmund A. Laport

When the actual value of degree-amperes on the vertical part of the antenna with a given power input to the system and the base current is all known, the unattenuated field strength at a unit distance can be computed directly. There is a linear relationship between actual degree-amperes A₀ and field strength as expressed by

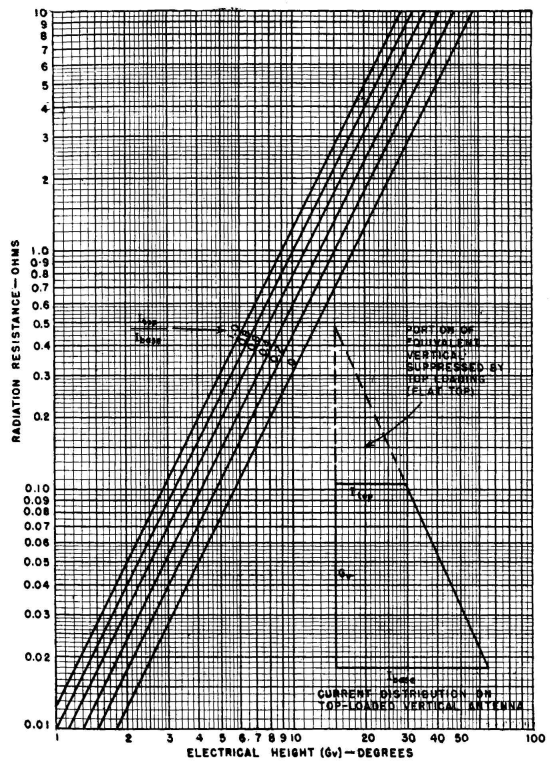
When F is the field strength in millivolts per meter at 1 mile, k = 0.650, and when F is given in volts per meter at 1 kilometer, k = 0.00104.

First Example. An example of the application of these relations is the solution of the following problem: A radiophare (or omnidirectional beacon) antenna for operation with low power on 200 kilocycles is desired. We can expect a total antenna resistance of the order of 3.5 ohms (judging from experience), and we want a radiation efficiency of about 15 percent. The radiation resistance of the antenna system therefore should be 0.53 ohm. By examination of Fig. 1.2 it is found that this resistance can be obtained by any of the realizable structures listed in Table 1.3.

FIG. 1.2. Radiation resistance for electrically short antennas versus height of vertical portion (degrees) and ratio of currents at top and base of vertical portion.

We therefore have to choose between one vertical radiator of 180 feet or two or more towers higher than 100 feet to support some form of top-loaded wire antenna. If the radiophare is near an airport, there may be objections to the 180-foot radiator as an obstruction. The 104-foot height takes a large amount of top loading to attain a ratio of 0.7 between currents at the top and base of the antenna. This creates additional mechanical loads, and more than two supports may be required.

Let us assume that we choose the 104-foot height in order to avoid the cost and complications of remotely controlling the transmitter. It is now desired to know what the fundamental frequency of the antenna must be.

Graphically, this is quickly solved by a diagram of height plotted against relative antenna current distribution. If we let 1.0 be the base current and 0.7 the current at 104 feet, a straight line drawn through these points and extended upward until it intersects with It/Ib = 0 will give the height of the equivalent vertical radiator with the same fundamental frequency. This height is found to be about 347 feet (25 degrees at 200 kilocycles). To get the required current taper on the 104-foot antenna the flat-top must simulate the capacitance of the upper 243 feet (17.3 degrees) of this equivalent vertical radiator.

A vertical radiator with a length of 25 degrees at 200 kilocycles would, by direct proportion, be 90 degrees at a frequency

The antenna under study must therefore be built to have a fundamental frequency of about 719 kilocycles for a vertical height of 104 feet.

If the power input to the system is to be 250 watts, the antenna current will be 8.47 amperes if the system resistance is 3.5 ohms. The vertical section of the antenna will then have a degree-ampere area of

The unattenuated field strength at 1 mile is

Second Example. As another example, let us compute the radiation resistance, radiation efficiency, and field strength of an antenna that has been constructed and has the following measured values at an operating frequency of 50 kilocycles:

Fundamental frequency 176 kilocycles

Physical height of vertical portion 450 feet

Electrical height of vertical portion 8 degrees at 50 kilocycles

Electrical length of entire antenna 25.5 degrees at 50 kilocycles

Measured resistance at 50 kilocycles 2.8 ohms

The current distribution on the vertical portion is computed from the fundamental frequency of 176 kilocycles. A straight vertical antenna for this frequency would be approximately and this equivalent system also has an electrical length of 25.5 degrees at 50 kilocycles.

Its current distribution would therefore correspond to 25.5 degrees of a sine wave as measured from the top end. The lower 8 degrees of this equivalent portion would correspond exactly to the vertical portion of the actual antenna under study. The top loading of the antenna is equivalent to the upper 945 feet (17.5 degrees) of the equivalent straight vertical antenna.

The ratio of top current to base current is

Now, referring to Fig. 1.2, we read (for a vertical height of 8 degrees and an It/Ib ratio of 0.7) a radiation resistance of 0.56 ohm. The radiation efficiency is therefore

With 100 kilowatts input, the antenna current would be 190 amperes. The degree-ampere area A₀ is 1,290, which produces an unattenuated field strength of 1.34 volts per meter at 1 kilometer.