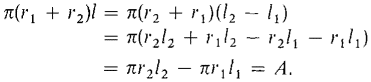| The ebook Elementary Calculus is based on material originally written by H.J. Keisler. For more information please read the copyright pages. |

|

Home  Applications of the Integral Applications of the Integral  Area of a Surface of Revolution Area of a Surface of Revolution  Cylinders and Cones Cylinders and Cones |
|






|
|
Cylinders and Cones
When a curve in the plane is rotated about the x- or y-axis it forms a surface of revolution, as in Figure 6.4.1.
Figure 6.4.1 Surfaces of Revolution The simplest surfaces of revolution are the right circular cylinders and cones. We can find their areas without calculus.
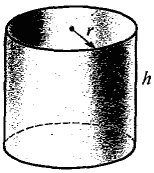
Figure 6.4.2 Cylinder Figure 6.4.2 shows a right circular cylinder with height h and base of radius r. When the lateral surface is slit vertically and opened up it forms a rectangle with height h and base 2nr. Therefore its area is lateral area of cylinder = 2πhr.
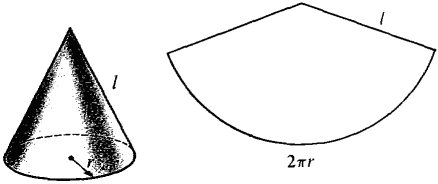
Figure 6.4.3: Cone Figure 6.4.3 shows a right circular cone with slant height l and base of radius r. When the cone is slit vertically and opened up, it forms a circular sector with radius l and arc length s = 2πr. Using the formula A = ½ sl for the area of a sector, we see that the lateral surface of the cone has area lateral area of cone = πrl.
Figure 6.4.4: Cone frustum Figure 6.4.4 shows the frustum of a cone with smaller radius r1, larger radius r2, and slant height l. The formula for the area of the lateral surface of a frustum of a cone is lateral area of frustum = π(r1 + r2)l. This formula is justified as follows. The frustum is formed by removing a cone of radius r1 and slant height l1 from a cone of radius r2 and slant height l2. The frustum therefore has lateral area A = πr2l2 - πr1l1. The slant heights are proportional to the radii,
The slant height l of the frustum is l = l2- l1. Using the last two equations,
|
|
Home  Applications of the Integral Applications of the Integral  Area of a Surface of Revolution Area of a Surface of Revolution  Cylinders and Cones Cylinders and Cones |
|
Last Update: 2010-12-07


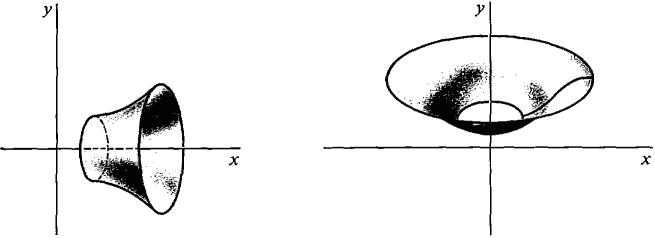

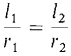 so r1l2 = r2l1.
so r1l2 = r2l1.