Validity of the Ideal Gas Law

Author: John Hutchinson

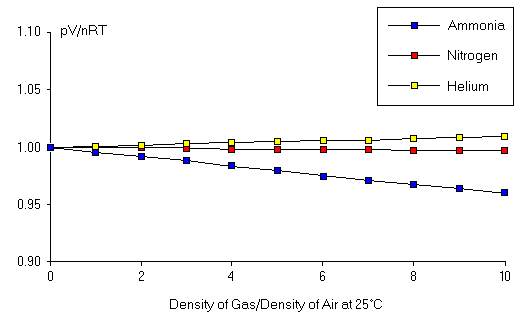
To design a systematic test for the validity of the Ideal Gas Law, we note that the value of PV/nRT, calculated from the observed values of P, V, n, and T, should always be equal to 1, exactly. Deviation of PV/nRT from 1 indicates a violation of the Ideal Gas Law. We thus measure the pressure for several gases under a variety of conditions by varying n, V, and T, and we calculate the ratio PV/nRT for these conditions.

Here, the value of this ratio is plotted for several gases as a function of the "particle density" of the gas in moles, n V . To make the analysis of this plot more convenient, the particle density is given in terms of the particle density of an ideal gas at room temperature and atmospheric pressure (i.e. the density of air), which is 0.04087mol L . In this figure, a particle density of 10 means that the particle density of the gas is 10 times the particle density of air at room temperature. The x-axis in the figure is thus unitless.

Figure 1: Validity of the Ideal Gas Law.

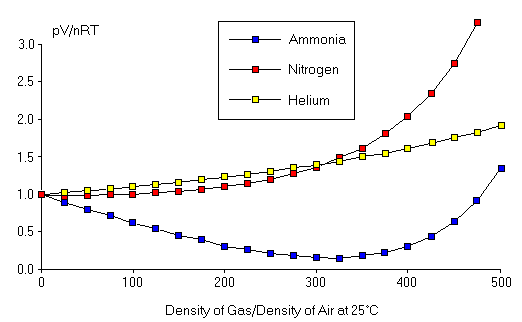
Note that PV/nRT on the y-axis is also unitless and has value exactly 1 for an ideal gas. We observe in the data in this figure that PV/nRT is extremely close to 1 for particle densities which are close to that of normal air. Therefore, deviations from the Ideal Gas Law are not expected under "normal" conditions. This is not surprising, since Boyle's Law, Charles' Law, and the Law of Combining Volumes were all observed under normal conditions. This figure also shows that, as the particle density increases above the normal range, the value of PV/nRT starts to vary from 1, and the variation depends on the type of gas we are analyzing. However, even for particle densities 10 times greater than that of air at atmospheric pressure, the Ideal Gas Law is accurate to a few percent.

Thus, to observe any significant deviations from PV=nRT, we need to push the gas conditions to somewhat more extreme values. The results for such extreme conditions are shown here. Note that the densities considered are large numbers corresponding to very high pressures. Under these conditions, we find substantial deviations from the Ideal Gas Law. In addition, we see that the pressure of the gas (and thus PV/nRT ) does depend strongly on which type of gas we are examining. Finally, this figure shows that deviations from the Ideal Gas Law can generate pressures either greater than or less than that predicted by the Ideal Gas Law.

Figure 2: Deviations from the Ideal Gas Law.