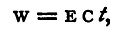Electrical Resistance

It is found by experiment that the ratio of the E.M.F. between two points to the current it produces, depends only on the con-ductor which connects the two points, and is called the resistance of the conductor.

The reciprocal of the resistance - that is, the ratio of the current to the electromotive force - is called the conductivity of the conductor.

Thus between any two points on a conductor there is a certain definite resistance : a metal wire, for example, has an electrical resistance of so many units depending on its length, cross-section, material, and temperature. Resistance coils are made of such pieces of wire, covered with an insulating material, cut so as to have a resistance of a certain definite number of units and wound on a bobbin. The ends of the coil are fastened in some cases to binding screws, in others to stout pieces of copper which, when the coil is in use, are made to dip into mercury cups, through which connection is made with the rest of the apparatus used. We refer to §78 for a description of the method of employing such coils in electrical measurements.

Standards of resistance have the advantages of material standards in general. The resistance is a definite property of a piece of metal, just as its mass is. The coil can be moved about from place to place without altering its resistance, and so from mere convenience electrical resistance has come to be looked upon as in some way the fundamental quantity in connection with current electricity. We have defined it by means of Ohm's law as the ratio of electromotive force to the current. Whenever difference of potential exists between two points of a conductor, a current of electricity is set up, and the amount of that current depends on the E.M.F. and the resistance between the points.

We may say that electrical resistance is that property of a conductor which prevents a finite electromotive force from doing more than a finite quantity of work in a finite time, Were it not for the resistance, the potential would be instantaneously equalised throughout the conductor; a finite quantity of electricity would be transferred from the one point to the other, and therefore a finite quantity of work would be done instantaneously.

The work actually done in time t is, we have seen,

and by means of the equation C = E/R expressing Ohm's law, we may write this

Moreover the E.M.F. between two points is given if we know the resistance between them and the current, for we have E = CR.