Core-Loss Current and Magnetizing Current

The fundamental, 3rd, and 5th harmonic components of the current in a reactor are shown in Fig. 5-6. Such a current could also be the exciting current or no-load current in a transformer. The fundamental component i_exc1 is shown in Fig. 5-7 along with the flux wave Φ and induced voltage wave e. Sinusoidal flux variation is assumed. In Section 5-4 it was shown that in the case of sinusoidal voltage the harmonics in the current wave do not contribute to the real power. Therefore, core loss, i.e., hysteresis and eddy-current losses, can be taken into account by taking the product of the induced emf and the component of the fundamental in the current that is in phase with the induced emf. This component of current is called the core-loss current and is represented by i_c in Fig. 5-7.

Figure 5-7. Core-loss current, fundamental component of exciting current, flux, and induced voltage waves

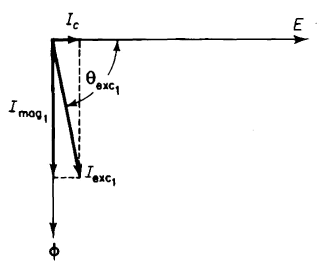
If the current wave representing the core-loss current ic is subtracted from the total fundamental component of current i_exc1, the result is a sine wave of current that is in phase with the flux wave Φ. If there were no harmonics, this latter component of current would be the magnetizing current. Under these conditions the two currents, i.e., core-loss and magnetizing currents, as well as the flux and the induced voltage, could be represented by the phasor diagram shown in Fig. 5-8. However, the magnitude of the exciting current is actually greater than that resulting from the fundamental component, as it does include all the harmonic components. Since the harmonics in the exciting current do not contribute to the real power if the induced voltage is sinusoidal, the core-loss component I_c in Fig. 5-8 is not affected by inclusion or exclusion of the harmonics. In accordance with Eq. 5-46, the exciting current has an rms or effective value of

[5-48]

It must be kept in mind that under a-c excitation, in the absence of a d-c component, only odd harmonics are present in the exciting current.

Figure 5-8. Phasor diagram of induced voltage, flux, core-loss current, and fundamental components of exciting current and magnetizing current

Figure 5-9. Phasor diagram including harmonics in the exciting current

The following relationship is obtained from Eq. 5-48

[5-49]

Generally, phasor diagrams apply to sinusoidal quantities, but may be used to include nonsinusoidal currents and voltages if these are replaced by equivalent sinusoidal quantities, i.e., sinusoids that have the same rms or effective values. If the exciting current is represented by a phasor I_exc as in Fig. 5-9 and is treated as an equivalent sinusoidal current, the phasor difference expressed by the right-hand side of Eq. 5-49 leads to a phasor that lags the core-loss current by 90°. The equivalent sinusoid represented by this phasor is called the magnetizing current, and from Eq. 5-49 is seen to be

[5-50]

Equation 5-50 suggests that the harmonic components be included in the magnetizing component of the exciting current. This facilitates the development of a phasor diagram and a simple equivalent circuit. A phasor diagram including the harmonics in the magnetizing current is shown in Fig. 5-9.

Example 5-1:	The following quantities were measured at no load on the 240-volt winding of a 30-kva, 2400/240-volt, 60-cycle transformer at rated frequency Volts = 240 Exciting current =2.53 amp Power = 164 w Determine the core-loss current and the magnetizing current. Neglect the resistance of the transformer winding.
Solution:	The exciting current Iexc = 2.53 amp, and